
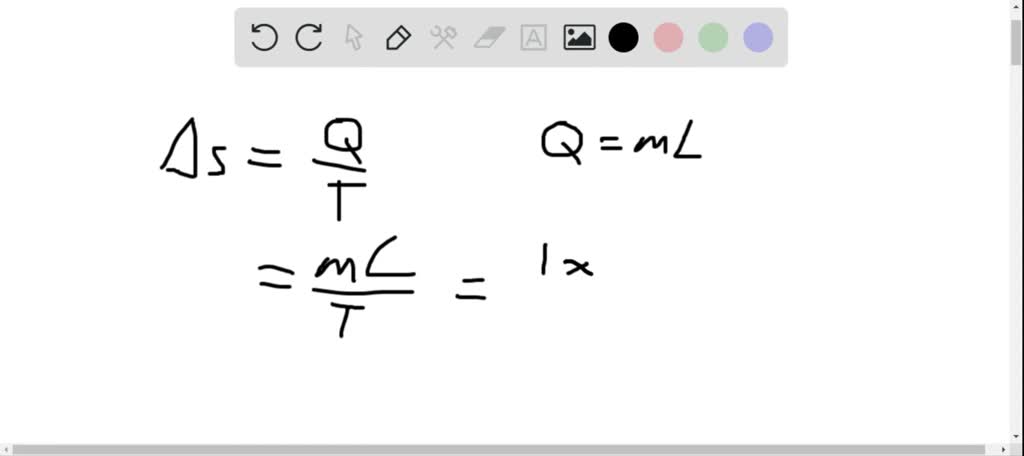
That is, the decrease in temperature during the irreversible expansion will be lesser and the increase in temperature during the irreversible compression will be larger that the corresponding change in the reversible process. Remembering that T 2 < T 1 in the expansion process and T 2 < T 1 in the compression process, we have T 2 < T 1
Where T 2 is the temperature, if the process was reversible, Where T 2 is the temperature of the system in the final state. 1, which is the information-theoretic definition of entropy. Clearly, the result of MICE will be an estimate of the entropy defined in Eq. In the present case of expansion (or compression), the increase (or decrease) in entropy due to the volume change just compensate the decrease (or increase) in entropy due to the fall (or rise) in temperature. In particular, the scheme presented above can be applied to out-of-equilibrium systems, whose entropy calculation is a challenge both technically and conceptually (8, 15, 17, 18, 39, 40). Since for the adiabatic reversible process, Now, we proceed to evaluate the change in total entropy for the following categories. Since in an adiabatic process, both temperature an volume (or pressure) change, the expression for the molar entropy change as given by Since magnitude of work done in the intermediate expansion is smaller than that involved in reversible expansion,Įntropy Change in Adiabatic Expansion or Compression of an Ideal GasĮntropy Change of System: Since in adiabatic processes q = 0, therefore Q rev = Heat absorbed by the system if the process had been carried out reversibly.įor irreversible expansion, work is done against constant pressure. Note: The entropy change of the system ΔS sys will be same in all three process as it is state function. It is because from surrounding no heat is supplied.
ENTROPY CALCULATOR FREE
Name: Worksheet: Entropy and Gibbs Free Energy In chemistry, the word spontaneous is used to describe Study Resources. View Copy of Entropy & gibbs free energy.pdf from CHEMISTRY 0014567 at Peach County High School.

Since entropy is a state function, the entropy change of a system in going from volume V 1 to V 2 by any path will same as that of a reversible change. Show the calculations that would need to be carried out to. Q rev is heat exchanged reversible between the system and the surrounding at temp T.Ĭase I : Free expansion: The gas expands into a vacuum for this process. Reversible Change: For reversible expansion or Compression.


 0 kommentar(er)
0 kommentar(er)
